Physicists Measure the Gravitational Force between the Smallest Masses Yet

Physicist Markus Aspelmeyer vividly remembers the day, nearly a decade ago, that a visitor to his lab declared the gravitational pull of his office chair too weak to measure. Measurable or not, this force certainly ought to exist. Ever since the work of Isaac Newton in 1687, physicists have understood gravity to be universal: every object exerts a gravitational force proportional to its mass on everything around it. The visitor’s comment was intended to bring an increasingly fanciful conversation back down to Earth, but Aspelmeyer, a professor at the University of Vienna, took it as a challenge. “My resolution was ‘Okay, I am going to not only measure the gravitational field of this chair, but we are going to go small, small, small!’” he recalls.

The research effort born on that day has now produced its first result: a measurement of the gravitational force between two tiny gold spheres, each about the size of a sesame seed and weighing as much as four grains of rice—the smallest masses whose gravity has been measured to date. The results, published in Nature today, bring physicists one step closer to the distant goal of reconciling gravity with quantum mechanics, the theory underlying all of nongravitational physics.

Precision Gravity

It is hard to fathom just how extraordinarily weak gravity is for such small masses. The gravitational pull of one sphere (the “source mass”) on the other (the “test mass”) a few millimeters away is more than 10 million times smaller than the force of a falling snowflake. The central challenge facing Aspelmeyer’s team was to design a detector exquisitely sensitive to this gravitational force yet totally insensitive to much larger background forces pushing and pulling on the test mass from all sides.

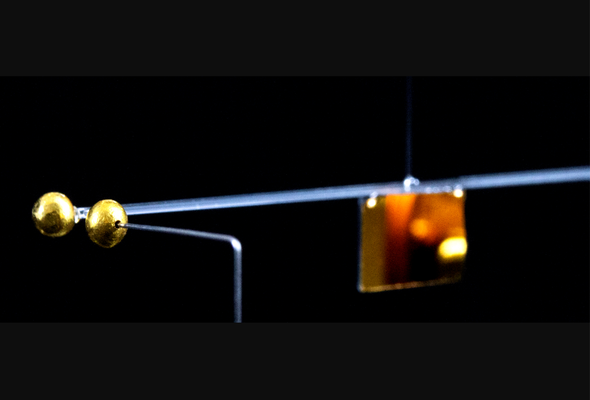
The researchers achieved this sensitivity using a detector called a torsion pendulum, which looks like a miniature version of a mobile hanging above a child’s crib. The test-mass sphere is fixed to one end of a thin rod that is suspended at its midpoint by a four-micron-thick quartz fiber. An identical sphere on the other end of the rod acts as a counterweight. A force on the test mass causes the torsion pendulum to rotate until it is balanced by a restoring force from the twisting of the fiber. Such a thin fiber is extremely compliant, so even a very weak force yields a relatively large rotation. Critically, the torsion pendulum is very insensitive to forces from distant objects, which tug on the test mass and counterbalance together and thus do not induce rotation.

But even this clever torsion pendulum design did not totally isolate the test mass from the busy urban environment of daytime Vienna. “The sweet spots are always between midnight and 5 A.M., when no people are on the street,” Aspelmeyer explains. “[But] this was not true of Friday or Saturday.”

To measure the gravitational force of the source mass, the researchers did not simply place it near the test mass. Instead they moved it continuously back and forth around an average separation of a few millimeters. This technique, called modulation, is implicit in the design of turn signals and blinking bike lights: regular, periodic signals are much more visible against ever-present background noise than constant ones. Sure enough, the scientists observed an oscillating force at precisely the right frequency. They then repeated this process many times, changing the average separation between the masses, and measured forces as small as 10 femtonewtons at separations between 2.5 and 5.5 millimeters. The team compared these measurements to Newton’s famous inverse square law of gravity, which describes how the gravitational force between two objects depends on their separation: the data were consistent with Newton’s law to within 10 percent.

“[That] you can measure these really, really, really tiny forces—I think that is pretty amazing,” says Stephan Schlamminger, a physicist at the National Institute of Standards and Technology, who studies gravity but was not involved in the work.

But Aspelmeyer and his colleagues could not declare victory quite yet: they still had to rule out the possibility that the source mass modulation was generating other forces on the test mass that would oscillate at precisely the same frequency. Periodic rocking of the table supporting the experimental apparatus, caused by recoil from the barely visible motion of the source mass, was just one of a host of confounders the researchers had to carefully quantify. In the end, they found that all known nongravitational forces would be at least 10 times smaller than the gravitational interaction.

Reaching toward Quantum Scales

Aspelmeyer believes that an improved torsion pendulum will be sensitive to gravity from masses 5,000 times smaller still—lighter than a single eyelash. His ultimate goal is to experimentally test the quantum nature of gravity, a question that has perplexed physicists for nearly a century. Quantum mechanics is one of the most successful and precisely tested theories in all of science: it describes everything from the behavior of subatomic particles to the semiconductor physics that makes modern computing possible. But attempts to develop a quantum theory of gravity have repeatedly been stymied by contradictory and nonsensical predictions.

Particles described by quantum mechanics behave in remarkably counterintuitive ways. One of the strangest kinds of quantum behavior is a special form of correlation called entanglement: when two particles become entangled, their fates become inextricably linked, and they cannot be described separately. Entanglement and other quantum effects are most prominent in very small and well-isolated systems such as atoms and molecules, and they become increasingly fragile on larger scales where gravity is relevant. Until recently, tests of quantum gravity have seemed far beyond the reach of laboratory-scale experiments.

But the past few years have seen remarkable experimental progress toward discerning subtle quantum effects in ever larger systems. In late 2017 two groups of theoretical physicists independently proposed an ambitious but possibly realizable experiment that could make a definitive statement about the quantum nature of gravity. The effort would measure whether gravity can entangle two quantum particles. If so, “there’s no escape from the fact that it has to be, in some sense, nonclassical,” says Chiara Marletto, a theoretical physicist at the University of Oxford, who co-authored one of the proposals with her Oxford colleague Vlatko Vedral.

The observation of gravitationally induced entanglement would be groundbreaking. But a conclusive demonstration that gravity is quantum mechanical would require proving that the two particles interacted only through gravity. Aspelmeyer’s efforts to isolate gravitational forces between progressively smaller masses are a critical step toward such a definitive test. “Since quantum is going from small to big, there’s a chance for gravity and quantum to meet somewhere in the middle,” says Sougato Bose, a theoretical physicist at University College London, who co-wrote the other proposal with nine collaborators.

“The question of whether gravity fundamentally behaves quantum is an experimental question,” Aspelmeyer says. “We can’t wait to go the whole nine yards and see how things turn out.”