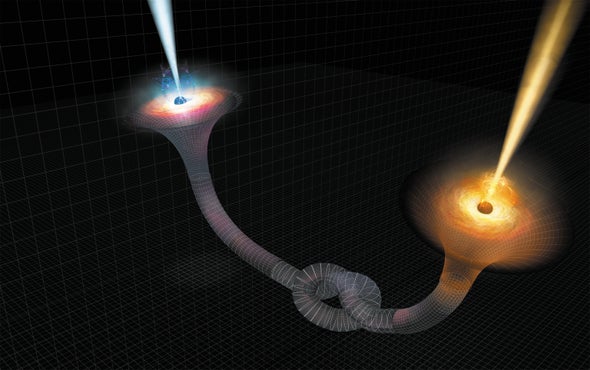
Theoretical physics is full of mind-boggling ideas, but two of the weirdest are quantum entanglement and wormholes. The first, predicted by the theory of quantum mechanics, describes a surprising type of correlation between objects (typically atoms or subatomic particles) having no apparent physical link. Wormholes, predicted by the general theory of relativity, are shortcuts that connect distant regions of space and time. Work done by several theorists, including myself, has suggested a connection between these two seemingly dissimilar concepts. Based on calculations involving black holes, we realized that quantum mechanics’ entanglement and general relativity’s wormholes may actually be equivalent—the same phenomena described differently—and we believe the likeness applies to situations beyond black holes.
This equivalence could have profound consequences. It suggests that spacetime itself could emerge from the entanglement of more fundamental microscopic constituents of the universe. It also suggests that entangled objects—despite having long been viewed as having no physical connection to one another—may in fact be connected in ways that are far less fantastical than we thought.
Furthermore, the relation between entanglement and wormholes may help in developing a unified theory of quantum mechanics and spacetime—what physicists call a theory of quantum gravity—that derives the physics of the macroscopic cosmos from the laws that govern the interactions of the atomic and subatomic realms. Such a theory is necessary for understanding the big bang and the interior of black holes.
Interestingly, both quantum entanglement and wormholes date back to two articles written by Albert Einstein and his collaborators in 1935. On the surface, the papers seem to deal with very different phenomena, and Einstein probably never suspected that there could be a connection between them. In fact, entanglement was a property of quantum mechanics that greatly bothered the German-born physicist, who called it “spooky action at a distance.” How ironic that it now may offer a bridge to extend his relativity theory to the quantum realm.
Black Holes and Wormholes
To explain why I think quantum entanglement and wormholes could be related, we must first describe several properties of black holes, which are intimately involved in this idea. Black holes are regions of curved spacetime that differ drastically from the relatively nondistorted space we are used to. The distinctive feature of a black hole is that we can separate its geometry into two regions: the exterior, where space is curved but objects and messages can still escape, and the interior, lying beyond the point of no return. The interior and exterior are separated by a surface called the event horizon. General relativity tells us that the horizon is just an imaginary surface; an astronaut crossing it would not feel anything special at that location. But having crossed it, a space traveler would be doomed to being squeezed into a region with huge curvature and with no possibility of escape. (In fact, the interior is actually in the future compared with the exterior, so the traveler cannot escape because he or she cannot travel to the past.)
Just a year after Einstein introduced general relativity, German physicist Karl Schwarzschild found the simplest solution to Einstein’s equations describing what would later be called black holes. The geometry that Schwarzschild came up with was so unexpected that it took until the 1960s for scientists to fully understand that it describes a wormhole joining two black holes. From the outside the black holes appear to be separate entities sitting at distant locations, yet they share an interior.
In a 1935 paper, Einstein and his colleague Nathan Rosen, then at the Institute for Advanced Study in Princeton, N.J., anticipated that this shared interior was a kind of wormhole (although they did not understand the full geometry it predicted), and for this reason wormholes are also called Einstein-Rosen (ER) bridges.
The wormhole in Schwarzschild’s solution differs from black holes that form naturally in the cosmos in that it contains no matter—it is merely curved spacetime. Because of the presence of matter, naturally formed black holes have only one exterior. Most researchers view the full Schwarzschild solution, with its two exteriors, as a mathematical curiosity irrelevant to the black holes in the universe. Nevertheless, it is an interesting solution, and physicists have wondered about its physical interpretation.
The Schwarzschild solution tells us that the wormhole connecting the two black hole exteriors varies with time. It elongates and becomes thinner as time progresses, like a piece of elastic dough being stretched. Meanwhile the two black hole horizons, which at one point touch, separate rapidly. In fact, they pull apart so quickly that we cannot use such a wormhole to travel from one exterior to the other. Alternatively we can say that the bridge collapses before we can cross it. In the dough-stretching analogy, the collapse of the bridge corresponds to the dough becoming infinitesimally thin as it gets stretched more and more.
It is important to note that the wormholes we are discussing are consistent with the laws of general relativity, which do not allow faster-than-light travel. In that way they differ from science-fiction wormholes that allow instantaneous transport between distant regions of space, as in the 2014 movie Interstellar. Science-fiction versions often violate the known laws of physics.
A sci-fi story involving our type of wormhole might look like the following. Imagine two young lovers, Romeo and Juliet. Their families do not like each other and so put Romeo and Juliet in different galaxies, forbidding them to travel. These lovebirds are very smart, however, and manage to construct a wormhole. From the outside the wormhole looks like a pair of black holes, one in Romeo’s galaxy and one in Juliet’s galaxy. The lovers decide to jump into the interior of their respective black holes. Now, according to their families, they simply committed suicide by jumping in and are never heard from again. Unbeknownst to the outside world, though, the geometry of the wormhole is such that Romeo and Juliet actually meet in the shared interior! And they can live together happily for a while before the bridge collapses, destroying the interior and killing them both.
Quantum Entanglement
The 1935 paper discussing the other phenomenon of interest to us—entanglement—was written by Einstein, Rosen and Boris Podolsky (also then at the Institute for Advanced Study). The three authors came to be known as EPR. In this famous work, the physicists argued that quantum mechanics allows for the existence of certain strange correlations between distant physical objects, a property that would only later be called entanglement.
Correlations between distant objects can also happen in classical physics. Imagine, for example, that you have a single glove with you because you forgot the other one at home. Before searching your pocket, you do not know whether you have the left or right glove. Once you see that you have the right-hand glove, though, you will immediately know that the one at home is the lefty. But entanglement involves a different kind of correlation, one that exists between quantities governed by quantum mechanics, which are subject to Heisenberg’s uncertainty principle. This principle says that there are pairs of physical variables that are impossible to know accurately at the same time. The best-known example involves the position and velocity of a particle: if we measure its position accurately, its velocity becomes uncertain, and vice versa. EPR wondered what would happen if we decided to measure either the positions or the velocities of the individual particles in a pair separated by a wide distance.
The example that EPR analyzed involves two particles with the same mass moving in a single dimension. Let us call these particles R and J because they are the particles that we will imagine being measured by Romeo and Juliet. We can prepare them in such a way that their center of mass has a well-defined position, which we will call xcm, equal to xR (the position of R) plus xJ (the position of J). We can require the center of mass to equal zero—in other words, we can say that the two particles are always equidistant from the origin. We can also make the particles’ relative velocity, vrel, equal to the velocity of R (vR) minus the velocity of J (vJ), take a precise value; for example, vrel equals some number we can call v0. In other words, the difference between the two velocities must stay the same. We are here specifying a position and a velocity accurately but not for the same single object, so we do not violate Heisenberg’s uncertainty principle. If we have two different particles, nothing prevents us from knowing the position of the first and the velocity of the second. Similarly, once we fix the position of the center of mass, we cannot say anything about the velocity of the center of mass, but we are free to fix the relative velocity.
Here we get to the most amazing part and the thing that makes quantum entanglement seem so strange. Suppose that our particles are far away from each other, and two distant observers, Romeo and Juliet, decide to measure the particles’ positions. Now, because of how the particles have been prepared, if Juliet determines any specific value for xJ, then Romeo will find that his particle’s position is the negative of Juliet’s (xR = xJ). Note that Juliet’s result is random: the position of her particle will vary from measurement to measurement. Romeo’s result, however, is completely fixed by Juliet’s. Now suppose they both measure their own particle’s velocity. If Juliet gets a specific result for vJ, then Romeo will surely find that his velocity is the value of Juliet’s plus the relative velocity (vR = vJ + v0). Again Romeo’s result is completely determined by Juliet’s. Of course, Romeo and Juliet are free to choose which variable they will measure. In particular, if Juliet measures the position and Romeo measures the velocity, their results will be random and will not display any correlation.
The strange thing is that even though Romeo’s measurements of the position and velocity of his particle are constrained by Heisenberg’s uncertainty principle, if Juliet decides to measure the position of her particle, Romeo’s particle will have a completely certain position once he knows the result of Juliet’s measurement. And the same thing will happen with the velocity. It appears as if, when Juliet measured the position, Romeo’s particle immediately “knew” that it must have a well-defined position and an uncertain velocity, whereas the opposite should be the case if Juliet measured the velocity. At first glance this situation appears to allow an instantaneous transmission of information: Juliet measures the position, and then Romeo sees a definite position for his particle, thus inferring that Juliet measured the position. Romeo would not be able to realize, however, that his particle has a definite position without knowing the actual value of the position that Juliet measured. So in fact, correlations caused by quantum entanglement cannot be used to send signals faster than the speed of light.
Although it has been experimentally confirmed, entanglement may still seem just an esoteric property of quantum systems. Yet during the past two and a half decades these quantum correlations have led to a number of practical applications and breakthroughs in fields such as cryptography and quantum computing.
Equivalence
How might our two very different, bizarre phenomena—wormholes and entanglement—be related? A further look at black holes points the way to the answer. The late Stephen Hawking showed in 1974 that quantum effects will cause black holes to emit radiation in the same way a hot object does—proving that the traditional wisdom that nothing can escape a black hole is too simplistic. The fact that black holes radiate implies that they have a temperature—a notion with important ramifications.
Since the 19th century physicists have known that temperature stems from the movement of the microscopic constituents of a system. In a gas, for example, temperature arises from the agitation of molecules. Therefore, if black holes have temperatures, one can expect that they also have some kind of microscopic constituents that collectively are capable of adopting various possible configurations, or so-called microstates. We also believe that, at least as seen from the outside, black holes should behave as quantum systems; that is, they should be subject to all the laws of quantum mechanics. In summary, when we look at a black hole from the exterior, we should find a system that can have many microstates, with the probability of its being in any of these configurations essentially equal for all microstates.
Because black holes look like ordinary quantum systems from the outside, nothing prevents us from considering an entangled pair of them. Imagine a couple of very distant black holes. Each has a large number of possible microscopic quantum states. Now imagine an entangled pair of black holes in which each quantum state in the first black hole is correlated with the corresponding quantum state of the second. In particular, if we measure a certain state for the first hole, the other hole must be in exactly the same state.
The interesting thing is that, based on certain considerations inspired by string theory (one approach toward a theory of quantum gravity), we can argue that a pair of black holes with their microstates entangled in this way (that is, in what might be called an EPR entangled state) would produce a spacetime in which a wormhole (an ER bridge) links the interior of both black holes. In other words, quantum entanglement creates a geometric connection between the two black holes. This result is surprising because entanglement, we thought, involves correlations without a physical connection. But the two distant black holes in this case are physically connected through their interior and brought close via the wormhole.
Leonard Susskind of Stanford University and I have called the equivalence of wormholes and entanglement “ER = EPR” because it relates the two articles that Einstein and his colleagues wrote in 1935. From EPR’s point of view, the observations near the horizons of each black hole are correlated because the black holes are in a state of quantum entanglement. From ER’s vantage point, the observations are correlated because the two systems are linked through the wormhole.
Now, going back to our Romeo and Juliet sci-fi story, we can see what the lovers should do to form an entangled pair of black holes to produce the wormhole. First they need to create many entangled particle pairs, similar to the ones discussed earlier, with Romeo possessing one member of each entangled pair and Juliet the other. They then need to build very complex quantum computers that will manipulate their respective quantum particles and combine them in a controlled way to create a pair of entangled black holes. Such a feat would be terribly hard to achieve in practice, but it seems possible according to the laws of physics. Besides, we did say Romeo and Juliet were very smart!
A Universal Principle?
The ideas that led us here have been developed over the years by many researchers, beginning with a 1976 article by Werner Israel, then at the University of Alberta. There was also interesting work in 2006 on the connection between entanglement and the geometry of spacetime by Shinsei Ryu and Tadashi Takayanagi, both then at the University of California, Santa Barbara. Susskind and I were motivated by research published in 2012 by Ahmed Almheiri, Donald Marolf, the late Joseph Polchinski and James Sully, all then at U.C. Santa Barbara. They discovered a paradox related to the nature of an entangled black hole’s interior. The ER = EPR idea, which says that the interior is part of a wormhole connecting the black hole to another system, alleviates some aspects of this paradox.
Although we identified the connection between wormholes and entangled states using black holes, it is tempting to speculate that the link is more general—that whenever we have entanglement, we have a kind of geometric connection. This expectation should hold true even in the simplest case, in which we have only two entangled particles. In such situations, however, the spatial connection could involve tiny quantum structures that would not follow our usual notion of geometry. We still do not know how to describe these microscopic geometries, but the entanglement of these structures might somehow give rise to spacetime itself. It is as if entanglement can be viewed as a thread connecting two systems. When the amount of entanglement becomes larger, we have lots of threads, and these threads could weave together to form the fabric of spacetime. In this picture, Einstein’s relativity equations are governing the connections and reconnections of these threads; quantum mechanics is not just an add-on to gravity—it is the essence of the construction of spacetime.
For now this picture is still wild speculation, but several clues point toward it, and many of us physicists are pursuing its implications. We believe that the seemingly unrelated phenomena of entanglement and wormholes might in fact be equivalent and that this equivalence provides an important clue for developing a description of quantum spacetime—and a long-awaited unification of general relativity and quantum mechanics.